Pseudorandom Number Generators
A simple discussion on randomising RGB lights in the QMK discord led me down the rabbit hole of pseudorandom number generators. The original goal was replacing C library’s rand() function with a smaller function to generate random unsigned 8-bit numbers for RGB. Experiments on a number of code sample showed that creating good RNG in 8-bit is not trivial. Documented here are noteworthy algorithms found on the Interweb.
Evaluating PRNGs
Visually with bitmap
While pseudorandom number generators are not of cryptography quality, we do want an algorithm that do not repeat themselves for animation and visual lighting use-cases. Rendering PRNG output as bitmap is a simple way to review for regular patterns and this simple bitmap code does that trick. Colours in the code loop array can be assigned the same uniform uint8_t output from a PRNG (prng_function()) to render a greyscale image:
for(int row = height - 1; row >= 0; row--) {
for(int col = 0; col < width; col++) {
uint8_t blue, green, red;
blue = green = red = (uint8_t)prng_function();
buf[row * width_in_bytes + col * 3 + 0] = blue;
buf[row * width_in_bytes + col * 3 + 1] = green;
buf[row * width_in_bytes + col * 3 + 2] = red;
}
}
The absence of pattern does not imply good output but bitmap image is a fun and quick way to detect poor one.
Empirically with PractRand
For more serious use of PRNG output, the simple PractRand tool tool can be used to evaluate output quality. Ideal PRNGs should only start failing at very large output test sizes. See this post for more details on setting up PractRand tests.
32 and 64-bit PRNGs
Large state space of 32 and 64-bit is where one can find many PRNGs. They are overkill for embedded systems like QMK that rarely need big random numbers and compiled code sizes are larger than rand(). Nonetheless if you want something bespoke, listed in this section are interesting ones that passes PractRand tests. It is worth noting that truncated uint8_t output of these codes are on par with its full length results. Hence they can also be visually rendered with unsigned RGB grey values.
PCG32
Melissa O’Neill published her paper and PCG (permuted congruential generator) family of codes at www.pcg-random.org. Her most robust PCG32 code has many versions—the following is a seeded version of 64-bit state with XORshift and random-rotation:
// pcg_mcg_64_xsh_rr_32_random_r
uint32_t pcg32(void) {
// Seed this 64bit manually
static uint64_t state = 0x406832dd910219e5;
uint64_t oldstate = state;
state = state * 6364136223846793005ULL;
uint32_t value = ((oldstate >> 18U) ^ oldstate) >> 27U;
uint32_t rot = oldstate >> 59U;
return (value >> rot) | (value << ((- rot) & 31));
}
Xoroshiro++
Shift-register generator using XORshift was first discovered by mathematician George Marsaglia. Weaknesses of earlier implementations were improved with XORshift and rotate, dubbed xoshiro / xoroshiro. The following is the general purpose and fast xoroshiro128++ using 128-bit state (with two uint64_t):
uint64_t rol64(uint_fast64_t const x, int const k) {
return (x << k) | (x >> (64 - k));
}
// xoroshiro128++
uint64_t xoroshiro128pp(void) {
// Seed both 64bit manually
static uint64_t s0 = 0xaafdbd4fce743b4d;
static uint64_t s1 = 0xcaee5c952c4ae6a8;
uint64_t const t0 = s0;
uint64_t t1 = s1;
uint64_t const result = rol64(t0 + t1, 17) + t0;
t1 ^= t0;
s0 = rol64(t0, 49) ^ t1 ^ (t1 << 21); // a, b
s1 = rol64(t1, 28); // c
return result;
}
16-bit PRNGs
These are exponentially smaller and is more practical for embedded firmware. Small state sizes strike a good balance of output quality versus code size and its output can be type-casted to unint8_t as needed. These will also fail PractRand tests on smaller output.
PCG16
The 16-bit output version of Melissa PCG code is robust enough to pass most of PractRand tests, failing at 2^30 bytes (1 gigabyte). This random-rotate version use a single 32-bit state and is a good code choice for this size:
// pcg_mcg_32_xsh_rr_16_random_r
uint16_t pcg16(void) {
// Seed this 32bit manually
static uint32_t state = 0x406832dd;
uint32_t oldstate = state;
state = state * 747796405U + 1U;
uint16_t value = ((oldstate >> 10U) ^ oldstate) >> 12U;
uint32_t rot = oldstate >> 28U;
return (value >> rot) | (value << ((- rot) & 15));
}
Do note that all versions of PCG store state sizes that are ‘twice’ the size of its output. Keep this in mind when deploying PCG on embedded systems.
xorshift16
Brad Forschinger shrank Marsaglia’s XORshift into the following simple 32-bit state (two uint16_t) random-shift version. It fails PractRand tests with just 2^16 bytes (64 kilobytes):
uint16_t rnd_xorshift_16(void) {
// Seed both 16bit manually
static uint16_t x = 1, y = 1;
uint16_t t = (x ^ (x << 5U));
x = y;
return y = (y ^ (y >> 1U)) ^ (t ^ (t >> 3U));
}
JSF16
Bob Jenkin shared his small PRNG that uses 128-bit state. Dubbed as ‘chaotic’ Jenkins Fast Small or JSF, it was adapted to different state sizes. Here is the 16-bit version using 64-bit internal state:
#define rot16(x,k) (((x) << (k))|((x) >> (16 - (k))))
uint16_t jsf16(void) {
static uint16_t a = 0xf1ea;
static uint16_t b = 0x80cc, c = 0x80cc, d = 0x80cc;
uint16_t e = a - rot16(b, 13);
a = b ^ rot16(c, 8);
b = c + d;
c = d + e;
return d = e + a;
}
Unlike its peers, this algorithm will only fail PractRand at a huge 2^47 bytes (128 terabytes!) output; making it a very good PRNG for large 16-bit samples.
8-bit PRNGs
This space is where limitation of state sizes become apparent. Poorly implemented linear-feedback shift register (LFSR) codes will render bitmap repetition and fail PractRand at low output sizes.
Tzarc’s XORshift
@tzarc’s version of XORshift was the start of this rabbit hole and his code follows:
uint8_t prng(void) {
// Seed this 8bit manually
static uint8_t s = 0xAA, a = 0;
s ^= s << 3;
s ^= s >> 5;
s ^= a++ >> 2;
return s;
}
It is a modified XORshift using two 8-bit state and is the smallest. However the output fails PractRand at 2^13 bytes (8 kilobytes) and produces vertical bitmap image lines:

PCG8
An 8-bit output version of PCG can be found in the pcg-c-basic library. It runs on 16-bit state with xorshift and random-rotation:
// pcg_mcg_16_xsh_rr_8_random_r
uint8_t pcg8(void) {
static uint16_t state = 0x2fd5;
static uint16_t const inc = 0x8893;
uint16_t x = state;
state = state * 12829U + (inc|1);
uint16_t value = ((x >> 5U) ^ x) >> 5U;
uint32_t rot = x >> 13U;
return (value >> rot) | (value << ((- rot) & 7));
}
State multiplication will be slower on small systems. Unlike its larger PCG cousins, this fails PractRand with just 2^10 bytes and its image output has visual waves:
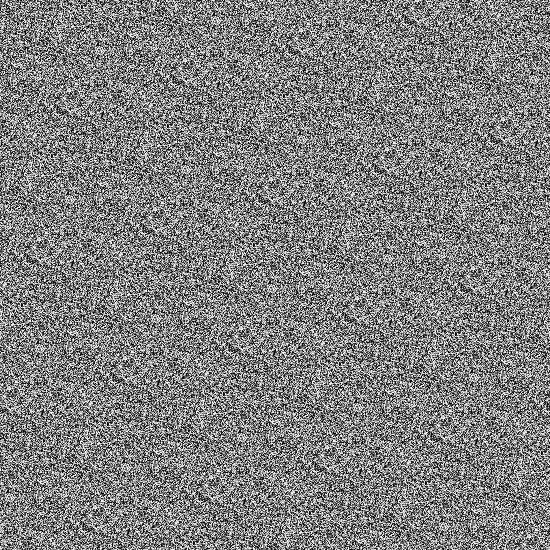
xshift8
Edward Rosten’s rng-4294967294 is a simple 32-bit state (four 8-bit registers) XORshift algorithm, using random-shift:
uint8_t xshift8(void) {
static uint8_t x = 0, y = 0, z = 0, a = 1;
uint8_t t = x ^ (x << 5);
x = y;
y = z;
z = a;
return a = z ^ ( z >> 1) ^ t ^ (t << 3);
}
His simple algorithm is surprisingly robust, failing PracRand at large 2^16 bytes (64 kilobytes) output and renders a pattern-free bitmap, making it a good 8-bit candidate for small systems:

JSF8
Finally there is Jenkin’s ‘chaotic’ PRNG, in the adapted 8-bit output version that uses rotation of 32-bit internal state:
#define rot8(x,k) (((x) << (k))|((x) >> (8 - (k))))
uint8_t jsf8(void) {
static uint8_t a = 0xf1;
static uint8_t b = 0xee, c = 0xee, d = 0xee;
uint8_t e = a - rot8(b, 1);
a = b ^ rot8(c, 4);
b = c + d;
c = d + e;
return d = e + a;
}
This diminutive algorithm is both small and fast; failing PractRand at a large 2^29 bytes (512 megabytes) output. Its bitmap output is also pattern free—making it the best 8-bit PRNG for embedded firmware:

QMK compile sizes
Here is a comparison of QMK firmware sizes for each PRNG compiled with LTO_ENABLE = yes:
| PRNG | Compiled results | Byte size |
|---|---|---|
| No PRNG | 10902 bytes free | NULL |
rand() | 10582 bytes free | 320 |
pcg32() | 10278 bytes free | 624 |
xoroshiro128pp() | 10110 bytes free | 792 |
pcg16() | 10622 bytes free | 280 |
rnd_xorshift_16() | 10786 bytes free | 116 |
jsf16() | 10736 bytes free | 166 |
prng() | 10802 bytes free | 100 |
pcg8() | 10738 bytes free | 164 |
xshift8 | 10790 bytes free | 112 |
jsf8() | 10796 bytes free | 106 |
Footnote
Init code for seeding states were not include in these samples—they are seeded manually because there is no random source in a QMK keyboard. Suffice to say that codes here should not be applied on sensitive cryptography use cases–they are ‘pseudorandom’ after all.